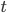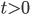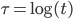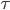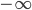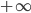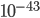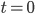Did the Universe Begin? X: Recapitulation

by Aron Wall Posted on October 21, 2014

from http://www.xkcd.com/1352/.

We have now come to the end of my series about whether or not the universe had a beginning.  This is part of a longer series dissecting the debate between St. William Lane Craig and Sean Carroll.  I started out with some general reflections on the debate:

Thoughts on the Carroll-Craig Debate
God of the Gaps  (see also: Gaps at the Dinner Table)

Then I started talking specifically about possible evidence from physics for and against the universe having a beginning.  For ease of understanding I'm going to label each main new argument with FOR or AGAINST to define its main orientation, but the posts also deal with the various counterarguments (that's the tire swing going back and forth above...). I've provided an executive summary of each of these posts, so that you can easily see the main thrust of what I said.  Minus all the caveats, hedging, and detailed explanations my scientific training tends to encourage.

(I've heard that politicians hate talking to scientists because, like the Elves in Tolkien, we seldom give a straight answer to a question.  In scientific cultures, we show "sincerity" by discussing all the problems and caveats with our ideas, whereas in political circles this sounds like insincere waffling designed to please too many people...)

Did the Universe Begin? I: Big Bang Cosmology (FOR, as far as it goes...)
- the classical Big Bang Model predicts an initial singularity where time began
- tentative because quantum effects were important and invalidate our usual geometrical notions
- also tentative because we don't really know how inflation began

Did the Universe Begin? II: Singularity Theorems (FOR)
- classical General Relativity theorems by Hawking and Penrose
- assumptions of Hawking theorem invalid during inflationary epoch
- Penrose theorem says that if space is infinite, there was a beginning
- Penrose theorem invalid in quantum situations, but my work suggests that it might be extendable to quantum gravity, if horizons always obey the 2nd law of thermodynamics.

Did the Universe Begin? III: BGV Theorem (FOR)
- if the universe has a positive average expansion, then "nearly all" geodesics cannot be extended infinitely to the past
- implies that inflation had to have a beginning in time, at least in some places
- can evade theorem by a "bouncing" cosmology where the universe contracts and then expands

Did the Universe Begin? IV: Quantum Eternity Theorem (AGAINST)
- if the usual rules of QM hold at all times, you can calculate what the state would be at any time to the past or future.
- in realistic cosmologies the energy is probably either zero or undefined, making the theorem inapplicable.

Did the Universe Begin? V: The Ordinary Second Law (FOR)
- given reasonable assumptions, 2nd law of thermodynamics requires a beginning
- most plausible way to evade this is to postulate that the "arrow of time" reverses
- such models would have a "thermodynamic beginning" but no "geometrical beginning"

Did the Universe Begin? VI: The Generalized Second Law (FOR)
- second law of thermodynamics also seems to apply to cosmological horizons
- can be used like ordinary 2nd law to argue for beginning
- can also be used as singularity theorem (see II above)
- this closes certain loopholes, but if the universe is finite and the arrow of time reverses, a bounce may still be possible.

Did the Universe Begin? VII: More about Zero Energy
- a more technical explanation of why the energy of the universe can be zero

Did the Universe Begin? VIII: The No Boundary Proposal (AGAINST/FOR)
- a beautiful set of speculative ideas which unify the "laws of physics" with the "initial conditions", by providing a rule for what the state of the universe is.
- contrary to popular conceptions, the Hartle-Hawking proposal has no beginning in time
- the Vilenkin tunnelling proposal is similar in spirit but does have a beginning.
- unclear whether these proposals are well defined, and Hartle-Hawking appears to give wrong predictions.

Did the Universe Begin? IX: More about Imaginary Time
- a more technical explanation about the notion of imaginary time used by Hartle-Hawking

If you put all of the physics information together, the conclusion I would draw is that: We don't know for sure whether the Universe began, but to the extent that our present-day knowledge is an indicator, it probably did.  However, as Carroll correctly says, we can also construct models where it doesn't have a beginning.  Taking into account known results from geometry and thermodynamics, the most plausible such models are 1) spatially finite, and 2) have a reversal of the arrow of time (e.g. the Aguirre-Gratton model).

I also noted that models like AG still have a low entropy "initial condition" somewhere in the middle of time.  One might think that this type of "thermodynamic beginning" still calls out for some type of explanation.

Then I wrote a more theologically-oriented post about whether the Hartle-Hawking no boundary proposal leaves any room for God to have created the universe:
Fuzzing into Existence
- short answer: yes, if you think of God as a storyteller, not a mechanic.

I also discussed the possibility of Reparameterizing Time; is it even meaningful to ask whether time is infinite or finite when you can change coordinate systems?  In this post I also argued that the main theological question of whether the universe needs an explanation seems to me much the same whether the universe has finite or infinite time.

Now, let me make another observation about the tire swing.  Although the weight of the evidence is that the universe probably had some sort of beginning—and even more likely that there was some sort of low entropy "initial condition" even if geometrically time stretches past before that—this cannot be said to be certain.  There is always the possibility that new scientific data or methods could radically change our picture of the very, very early universe.  Similarly, while a finite past seems more in accordance with traditional Christian theology than an infinite past, there appears to be no strictly logical connection between the two ideas, once the act of Creation is viewed in a more timeless, "authorial" way.  Thus one might conceivably have a theist who thinks time is infinite, or an atheist who thinks time was finite.

Should the argument for God's existence really rest on such a slender foundation as the ultimate decision of physicists about Big Bang Cosmology?  Well, one thing is clear.  In ages past it didn't depend on it.  Obviously, Sts. Abraham and Sarah, David and Solomon, the prophets and apostles, and all the men and women who followed in their footsteps up through the 19th century, including eminent scientists such as St. Faraday and St. Maxwell: these cannot have believed in God because of the Big Bang Theory, because—guess what?—nobody knew about it yet!  What does the Bible say about these people?

Now faith is confidence in what we hope for and assurance about what we do not see.  This is what the ancients were commended for.  By faith we understand that the universe was formed at the word of God, so that what is seen was not made out of what was visible.  (Hebrews 11:1-3)

Our belief that God is the Creator does not depend on the vicissitudes of scientific progress, the swinging back and forth of the tire swing (or is it accelerating?)  It doesn't matter, because in this case we have a more certain source of knowledge than Science.

By faith!  The skeptic may scoff here, and say that faith is belief without evidence, but that is not the definition used in the passage above.  It says that faith is confidence about what we hope for, but do not see.  Unless we identify sight (conceived broadly as anything which can be directly experienced in terms of our 5+ senses) with evidence (things which allow us to conclude something about the world)—an identification which would incidentally also make Science impossible—the passage does not say that the ancients were commended for believing without evidence.  But the example of the biblical heroes does give some pointers about what type of evidence was relevant to them.

The ancients did not believe that God was the Creator because they had a detailed scientific theory about where it comes from.  (Indeed, if we take our minds off Genesis for a moment and read the Wisdom literature of the Bible: Job and Psalms and Ecclesiastes and Proverbs, the Scriptures seem to emphasize more our lack of knowledge about the details of creation, then any detailed programme of events...)  On the contrary, the ancient Jews and Christians knew God, by personal acquaintance as it were, and therefore knew him to be creative and powerful, mighty in word and deed.  Thus they could take him at his word that he is the Creator of all that we see.

The glory of Creation does indeed point to the glory of the Creator, so that it is possible for ordinary human reasoners to come to know that there is a Creator intellectually.  But this sort of Theism, by itself, isn't what Christians mean by faith.  Once we come to know God personally, we learn the more important fact that we can trust him, and know with confidence that there is nothing in existence which does not depend on him.

And therefore, although we see in this world visible things emerging from other visible, material things, we know that ultimately their origin comes from "God’s invisible qualities—his eternal power and divine nature" (Rom 1:20).  He created everything through his Word, Jesus Christ, from whom we have come to know what God is like.  This way of knowing does not seem to depend very strongly on the details of past, present, or future scientific knowledge.

One could definitely argue that the Bible teaches that there was a Beginning (whatever this means from God's perspective).  For example, the quotation above from Hebrews speaks of the formation of the visible universe.  But whether or not this fact has been revealed by God, it is not obvious to me that the most important theological aspects of Creation really depend essentially on time being finite, or even well-defined.  (Admittedly, if you believe that time is infinite, it might be easier to slip into a false notion whereby matter exists independently of God, who is merely the Chief Organizer of the cosmos.  That would be a heresy—a false belief which may seriously obstruct your ability to relate to God or others properly—but it does not follow necessarily from time being infinite.)

The main point of the doctrine of Creation, I think, is that God is real, and that everything else is derived from his power and will.  We know this doctrine is true because we know God.  Not because of the Big Bang, as natural as it is to connect the two ideas.

Posted in Physics, Reviews, Theological Method, Theology | 50 Comments

Different Views about Time

by Aron Wall Posted on October 15, 2014

Updated to ask readers more directly for their thoughts, if you have any...

A random thought.  Suppose we ask whether the world has a Beginning or an End, or whether it is eternal is one or both directions.  It seems like there are 5 possible views, which I will name by association to various cultural groups who supposedly have had these views:

  1.  Norse view: the world began, and it will end.
  2.  Greek view: time is infinite in both directions
  3.  Hindu view: time goes in a circle
  4.  Hebrew view:  the world began, but it will never end.
  5.  Nobody ever: time had no beginning, but it will still end!

I find it interesting that the first four views all have some intuitive appeal, to different people, but the fifth view just seems horribly wrong and perverse!  Why do you suppose that is?

My best guess is that there's is a certain obvious symmetry to treating the past and future in the same way, which makes views (1-3) seem reasonable.  And there is also an argument that the past is not like the future, but if so it had better be like (4) rather than like (5)!  I guess we all know deep down (it's really the Second Law of Thermodynamics) that it makes sense for the universe to start from a simple initial condition and then develop complexities from there.  But if we have to deal with infinite regresses AND we don't even get an eternal universe out of it, that seems a bridge too far... but if anyone has any further thoughts on this, I'd be interested.

My cultural names are a brutal oversimplification, and you shouldn't take my assigning these views to different cultures too literally.  For one thing, there were lots of different Greeks and there are lots of different Hindus who believe all sorts of different things.  For another, there is a conceptual difference between the world—in the sense of an ordered cosmology with a history—beginning, and time (a much more abstract notion) having a beginning. It takes a certain amount of intellectual sophistication to think about the latter question.

Norse mythology begins with fire and ice swirling around a bottomless pit for aeons; it is only later that a bit of fire strikes a bit of ice and spontaneously generates a giant and a cow, from whom later the jotun and gods emerge by various removes.  (As you can see, the Norse were ultimately Materialists even about their so-called divinities.)  At the end, the cruel jotun defeat the merry gods and the world is destroyed, plunging back into chaos.  So it's not really clear that time has a beginning or end, just that the story has a beginning and an end.

The Hebrews had the notion of divine Creation in Genesis 1:1 and elsewhere, but it is controversial whether Genesis 1:1 actually teaches the creation ex nihilo of later theology.  St. Augustine is usually credited with the idea that there was not even time before creation, but in fact Philo, a 1st century Hellenistic Jew, got there first.

Similarly, our current best "concordance cosmology" appears to begin with an initial singularity, but has no end in time.  (Well, really we should talk about spacetime, which allows time to end in some places, e.g. inside black holes, but not others.)  This appears at first sight to be like the Hebrew view.  At late times the universe expands exponentially forever, thinning matter out to a very cold but finite temperature.  This is in accordance with the Generalized Second Law of Thermodynamics, which tells us that the universe will reach a boring maximum entropy state at late times.   Thus, the story ends at finite time, and we really have the heroic defiance against inevitable destruction, as in the Norse view.

Even in Hebrew cosmology, there is that little matter of the whole universe being destroyed and then recreated again:

“See, I will create
new heavens and a new earth.
The former things will not be remembered,
nor will they come to mind.
But be glad and rejoice forever
in what I will create,
for I will create Jerusalem to be a delight
and its people a joy.
I will rejoice over Jerusalem
and take delight in my people;
the sound of weeping and of crying
will be heard in it no more.

Never again will there be in it
an infant who lives but a few days,
or an old man who does not live out his years;
the one who dies at a hundred
will be thought a mere child;
the one who fails to reach a hundred
will be considered accursed.
They will build houses and dwell in them;
they will plant vineyards and eat their fruit.
No longer will they build houses and others live in them,
or plant and others eat.
For as the days of a tree,
so will be the days of my people;
my chosen ones will long enjoy
the work of their hands.
They will not labor in vain,
nor will they bear children doomed to misfortune;
for they will be a people blessed by the Lord,
they and their descendants with them.
Before they call I will answer;
while they are still speaking I will hear.
The wolf and the lamb will feed together,
and the lion will eat straw like the ox,
and dust will be the serpent’s food.
They will neither harm nor destroy
on all my holy mountain,”
says the Lord.  (Isaiah 65:17-25)

It's really this "new heavens and new earth" that will last forever.  Christianity is about Death and Resurrection, both for the universe and for each person.  Science can get us as far as the doomed-to-die bit, but it can't get us any farther.  That is Law, the rest is Grace, revealed in Jesus Christ.

Posted in Metaphysics, Scientific Method, Theological Method | 3 Comments

Reparameterizing Time

by Aron Wall Posted on October 14, 2014

In recent posts I've been discussing whether the universe began or not.

Perhaps the most important issue which I have not yet discussed, is the idea (I think originally due to Charles Misner, first pointed out by St. Edward Arthur Milne, and independently by St. Charles Misner) that it may not be well-defined whether time has a beginning or not.  That is, suppose you have a model in which there is a time coordinate t, and time has a beginning in the sense that the only allowed times are t > 0.  Well, in General Relativity we are free to use whatever time coordinate we like, and nothing stops us from defining a new time coordinate in terms of the old one, let's say \tau = \log(t).  If you look at a plot of the log function, you'll see that \tau ranges from -\infty to +\infty.

However, this type of time reparameterization may not be very physical once you get down to the Planck time, about 10^{-43} seconds, when quantum gravity effects become important.  Times less than that might not be well-defined.  In any case, the Misner argument suggests that we need to be more careful to define what we mean by time having a beginning.

Similarly, atheist philosopher Quentin Smith has argued that the standard Big Bang Model is inconsistent with divine creation, due to it not really having a beginning, even though the past is finite.  Smith argues that because the time t = 0 is singular, technically it shouldn't be included in the spacetime, so actually only times with t > 0 exist.  That means that there is no initial moment of creation, and therefore, he claims, God cannot have created the universe.

This is somewhat reminiscent of Hawking's claim that the no boundary proposal doesn't have the right sort of beginning, and it seems to me that my Fuzzing into Existence post is also applicable.  If God is like an author, then he can make a story in which time works in whatever way he pleases.

According to Smith, each time t exists because the preceding times exist, and indeed the laws of physics hold at a given time t (according to him) because they hold at earlier times.  Since each moment of time is fully explained by those before, he claims that the universe is therefore self-caused and therefore fully explained, with no more explanation possible.  (Of course, if time is continuous, then we could make a similar infinite regress of times going back closer and closer to any finite time t.  Smith has to struggle a bit to explain why his argument doesn't apply there...)

Now to me, this seems like the sort of explanation which is really no explanation at all.  A satisfying worldview should explain as much as possible with as few assumptions as possible.  If the laws of physics have some property X (e.g. having an electron field, or whatever) now because they were like X a minute ago, and so on all the way back arbitrarily close to the beginning, that doesn't in any way satisfy my curiosity about why they are like X instead of some other way Y (say, having no charged particles).  For if they had been Y for all time, I could have made the same argument.  So it seems that there is a potentially meaningful question "Why are the laws of physics like X rather than like Y", which Smith's statements do not really explain.  Maybe there is no explanation, and we have to take X being the way it is as a fundamental fact.  But to say that there could not possibly be an explanation seems rather dogmatic.

And if God exists, then he can explain this fact.  God's will chooses what the laws of physics will be for all time.  So he can choose for the universe to be like X instead of like Y.  This would be the fundamental explanation.  Whether or not it is a useful explanation for us as human beings, would depend on whether our puny minds can identify the actual reasons why God might prefer X over Y.

The Kalam argument has some intuitive appeal if you think that the universe could not have begun without some causal reason.  Evaluating this claim requires an analysis of what causation is, and why one would think in various situations that a cause is necessary.  But the first preliminary question is whether there are any facts to be explained by the putative cause.  It seems to me that there are.

All of the same reasoning about X and Y would also apply if time stretches back to -\infty.  There would still be various timeless facts about the universe which would not really be explained by the infinite regress.  This suggests that the Kalam argument may be misguided to the extent that it attempts to prove God from a temporal beginning a finite time in the past.  The most important issues are the same whether time goes back finitely or infinitely.

But having said all this, it does seem a little bit weirder that the universe should exist for a finite amount of time with no external explanation, than that it should exist for an infinite time with no explanation.   Historically, many materialists (such as Lucretius) have believed that time is infinite, due to their belief that it is impossible for something to come from nothing.  Conversely, monotheists have mostly believed that the universe has a beginning, either for philosophical reasons or because the Bible says so.  (St. Thomas Aquinas argued that God could have created an infinite past, but that divine revelation tells us he didn't.) To that extent, Big Bang cosmology appears to vindicate the standard religious view over the standard nonreligious one.

(Of course, the same cannot be said if—unlike St. Thomas or St. Augustine—one also takes the 6 day creation about 6,000 years ago literally.  Some fundamentalists have argued that this problem can be solved by reparameterizing our coordinate system, but that just seems silly to me.  Also, the days are not in the right order to correspond to the scientific chronology.)

But a Theist could believe that God created time going back infinitely, without contradicting themselves, so long as they are prepared to be flexible about what "creation" means.  Similarly, an Atheist could believe that the universe just started existing 13.8 billion years ago for no reason, without contradicting themselves, so long as they are prepared to be flexible when deciding when explanations are called for.  All four views are logically consistent; the real question is which viewpoint explains the most with the least.

Posted in Physics, Reviews, Theology | 8 Comments

Portuguese translations

by Aron Wall Posted on October 4, 2014

I'm pleased to announce that some of my blog posts have been translated into Brazilian Portuguese.  St. Felipe of Olhar Unificado (added to blogroll) has translated the following posts:

Castidade: Não é apenas para religiosos

Deus das Lacunas

O Teorema da Eternidade Quântica

Um Universo do Nada?

Apresentando a Buracos Negros #1 - Relatividade Especial
Apresentando a Buracos Negros #2 - Relatividade Geral
Apresentando a Buracos Negros #3 - Buracos Negros

Os Pilares da Ciência - Introdução
Os Pilares da Ciência #1 - Observação Repetida
Os Pilares da Ciência #2 - Hipóteses Elegantes
Os Pilares da Ciência #3 - Modelos Aproximados
Os Pilares da Ciência #4 - Descrições Precisas
Os Pilares da Ciência #5 - Integridade Ética
Os Pilares da Ciência #6 - Exame da Comunidade
Os Pilares da Ciência - Sumário [abridged]

Depressão

If you can't tell which posts of mine these are translations of, then this new feature might not be for you!  Felipe also has a bunch of his own writing on Science and Religion on Olhar Unificado, which I have added to my sidebar, but I can't say much about it, other than that it appears to be composed of words relevant to the topic.  There are, however, pictures.

Regarding the post on chastity, I don't know how it reads to someone who actually understands Portuguese, but just looking at the words, my talk about sex and romance seems ever so much more impressive in a Romance language!

As Felipe comes out with more translations, I will update the list on this post (without making any other announcement).  Thanks so much to Felipe for his endeavers.

Posted in Blog | 1 Comment

Moving to the Institute for Advanced Study

by Aron Wall Posted on August 27, 2014

In case you are wondering why I haven't been posting very much recently.... My postdoc at UC Santa Barbara ends this month, and on Sept 1st I will be starting an exciting new postdoc at the Insititute for Advanced Study near Princeton, as announced earlier.  I'm looking forward to some great conversations with people at the IAS and at Princeton.

So I've been rather tied up with finishing projects and packing books, not to mention two physics workshops I went to at McGill and at U British Columbia.  If there's ever a time when one is tempted to forswear possessions all together, it's when one is trying to clear out an apartment for a move.  Maybe we forget sometimes how practical the advice of Jesus is, to store up our treasures in Heaven rather than on Earth.

Really, it's kind of surprising I posted even once this month.  Hopefully things will be more peaceful once I arrive at Princeton, at least before all my stuff catches up with me!

Posted in Blog | 3 Comments