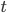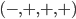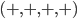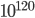In the last couple of posts, I've discussed the Hartle-Hawking proposal and the math behind it. Now let's discuss the theological implications.
In his Brief History of Time (written 1988; I'm just going to be engaging with this book and not with any of his more recent pronouncements), Hawking has the following famous saying about the Hartle-Hawking state:
The idea that space and time may form a closed surface without boundary also has profound implications for the role of God in the affairs of the universe. With the success of scientific theories in describing events, most people [!] have come to believe that God allows the universe to evolve according to a set of laws and does not intervene in the universe to break these laws. However, the laws do not tell us what the universe should have looked like when it started—it would still be up to God to wind up the clockwork, and choose how to start it off. So long as the universe had a beginning, we could suppose it had a creator. But if the universe is really completely self-contained, having no boundary or edge, it would have neither a beginning nor end: it would simply be. What place, then, for a creator?
The first question to ask here is who counts as "most people"?
The majority of people in the world believe in some type of God or gods capable of supernatural intervention. Even in the Western world, the majority of people believe in God (as Hawking indicates), and the majority of those believe in a religion called Christianity which teaches that God does produce miracles from time to time.
If Hawking means the English or the Europeans, then admittedly there has been a marked decline in religious faith in Europe (much less so in the US) and many "Christians" there have a merely nominal or cultural affiliation. But belief in miracles is still far from nonexistent.
In any case, I am obviously not the target demographic, since I believe that God has done some remarkable things since that moment, perhaps 13.8 billion years ago, when he set the ball rolling. Or was there such a moment?
Hawking suggests that (if his model is correct) there was no such moment of creation. Not, according to him, because the universe goes infinitely far back in time—he says that it doesn't. Rather, because the geometry of spacetime is rounded off like a sphere, so that there is no special beginning point, but rather a whole region of points none of which would be any better or worse as a beginning. As he says:
The universe would be completely self-contained and not affected by anything outside of itself. It would just BE.
Now this only works if you go to imaginary time to describe the universe. With respect to real time, the Hartle-Hawking state does go back forever in time (with high probability). So if real time is what is important, then what Hawking says about the absence of a beginning is still true, although for a different reason.
If the Hartle-Hawking proposal is right, this could itself be taken as good reason to endorse an "imaginary time" view of the universe, although I'm not sure that's a consistent thing to do given that we at any rate seem to live in real time. But Hawking himself expresses a more ambivalent view:
So maybe what we call imaginary time is more basic, and what we call real is just an idea that we invent to help us describe what we think the universe is like. But, according to the approach I described in Chapter 1, a scientific theory is just a mathematical model we make to describe our observations: it exists only in our minds. So it is meaningless to ask: which is real, "real" or "imaginary" time? It is simply a matter of which is the more useful description.
Yet on this more positivistic view where the model is only aiming to be a "useful description", how could one use it to draw the metaphysical deductions Hawking wants to make, about there being no "place" for a Creator? But let's leave that aside, and accept the "imaginary time" point of view for purposes of our theological excursion, since it doesn't much matter whether the universe lacks a beginning because it's closed off like a sphere, or because it goes back in time forever.
Now when Hawking asks rhetorically whether there is a "place" for a Creator, the context suggests that he's not so much asking whether there's good reason to believe in a Creator, but whether there even could be a Creator, given the absence of a clear first moment of time. What would there be left for him to do? Aside from deciding that there should be a universe, selecting the laws of physics for said universe, deciding that the Hartle-Hawking state is the prettiest state for it to be in, and then (according to Hawking) deciding not to intervene even if it turns out we could use some help. Other than that, it seems like there is nothing left for God to do!
Really, Hawking is assuming (quite explicitly) that Science has already displaced God to such an extent that the only "place" that could be left for him is to push the button to make everything go, and then "sit back and watch". (This view is often called Deism nowadays, although historically Deists actually had a much more robust view of divine providence, and merely rejected the miracles and special revelations of particular religions.)
This rather limited God is the type of bad theology which makes religious people throw around the phrase "God of the Gaps", although I still believe that this term is highly misleading and should be retired. I tried to express a better set of points in that post:
1. Any time we ever believe in anything rationally, we do so because there is some kind of "gap" in our understanding of how the universe works, which is filled by postulating the existence of that thing.
2. All phenomena which occur in Nature do so because God sustains the world in being, thus (at least indirectly) causing everything.
Hawking allows no role for God as the Sustainer of all existence. But God's role in "sustaining" the world is not really a different type of act from his act of "creating" it. Hawking invites us to look at the world from a 4-dimensional perspective; in this perspective all points of spacetime exist because God gives them the power to exist, delineating the role that each one plays in the bigger scheme of things. From that perspective, Creation is something which is happening NOW, not just something which happened (or didn't happen) 13.8 billion years ago. Stated in a tenseless way, for all the things that exist, they exist because God chooses for them the conditions of their existence. (One of those conditions being that they are causally related in particular ways to the events before, after, or around them.)
God's role in creation is not a "mechanical" one, providing the initial impetus or force to get the machine working, which can then run for a while on its own. God is more like an Author writing a story. An Author stands outside the time-stream of their own story. As my Dad said in a Slashdot interview:
Once you see the universe from that point of view, many arguments fade into unimportance, such as Hawking's argument that the universe fuzzed into existence at the beginning, and therefore there was no creator. But it's also true that the Lord of the Rings fuzzed into existence, and that doesn't mean it doesn't have a creator. It just means that the creator doesn't create on the same schedule as the creature's.
If God is creating the universe sideways like an Author, then the proper place to look for the effects of that is not at the fuzzy edges, but at the heart of the story. And I am personally convinced that Jesus stands at the heart of the story. The evidence is there if you care to look, and if you don't get distracted by the claims of various people who have various agendas to lead you in every possible direction, and if you don't fall into the trap of looking for a formula rather than looking for God as a person.
To think that God creates the universe and then stands back to watch it, is like thinking that an Author only has to write the first sentence, and then they can read the rest. Bad news for aspiring fiction writers: you have to write the whole thing. Maybe once the plot gets into full swing, the characters will start having a "mind of their own", and fail to act in the way the Author originally intended. But the Author is still in charge.
Nor does he have to "intervene" in order to get things to come out the way he wants them to: everything in the book is subject to the control of the Author, both the parts which follow naturally and inevitably from the previous scenes, and the parts where the Author does something totally unexpected. In any case, the main "point" of the story is seldom found right at the beginning, but develops as the story progresses.
Traditionally, books have a fixed and determinate sequence of letters, but if the Author wants to start out with something which doesn't have a definite time order (say a map on the first page) then that doesn't impugn their authorship of the rest of the book. And if the Author wants to make their book be infinitely long in both directions....well, that would probably be easier for God than for a human writer, wouldn't it!
So I think that belief in the creation of the universe does not really depend on there being a first moment of time. Conversely, this might also make one suspicious of the kalam argument championed by St. William Lane Craig in the debate. If the doctrine of Creation is not about there being a first moment of time, then there's something dubious about arguing for it as though it were. This doesn't automatically imply that St. Craig's argument is unsound, but it does suggest that it might not be the best way of looking at things.
Of course, we should also keep in mind what I said in my original post, that the Hartle-Hawking proposal is a speculative idea. It is a very beautiful idea, but it is difficult to make well-defined, and there is no direct evidence for it. While there was originally some reason to think it might predict inflation, the current indications seem to be that it predicts the wrong type of universe.
I remember my surprise when, several years ago, I read an article by the atheist philosopher Quentin Smith, showcasing the Hartle-Hawking state as an argument for Atheism. Never mind his actual argument, which makes no sense. In a talk given to some atheist club, he stated that his argument "is the strongest scientific argument there is against theism. I think it's even stronger than Darwin's theory of evolution."
Oh my! Neither Stephen Hawking nor Jim Hartle would make the claim that the Hartle-Hawking state is anywhere near as solidly supported as Darwinian evolution; in fact Jim told me just the other day that he isn't particularly committed to it being true. (People often assume that if a scientist thinks of an interesting, publishable idea, they must believe in it, but they might only think it is worth considering!) In fact, I think that only an outsider to the field of quantum gravity could take the "no boundary proposal" as anything other than a provisional, interesting idea worth exploring, which at best might be true.
I've discussed a lot of speculative physics in these last several posts, and I wouldn't want anyone walking away thinking that the physics is more clearly established than it is. In our current state of knowledge, any statements about the beginning of the universe are necessarily speculative, and if we rest our theological beliefs (for or against Theism) on that shaky foundation, we are setting ourselves up for trouble.

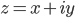
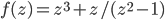
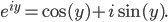

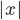
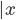
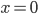
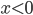
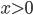
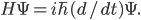
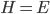
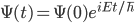

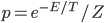
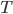
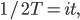
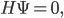
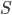
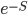
![^{[citation\,needed!]}](http://www.wall.org/~aron/blog/wp-content/plugins/latex/cache/tex_5bcaab41e3170d86c9052a23fde1103a.gif)