Previously, I described the main formula of Special Relativity:
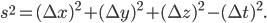
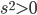 ) or the amount of duration squared (if
) or the amount of duration squared (if 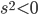 ). (By using some trigonometry we can also use this formula to figure out the size of angles, so this encodes everything about the geometry). All the crazy time dilation and distance contraction effects you've probably heard about are encoded in this formula.
). (By using some trigonometry we can also use this formula to figure out the size of angles, so this encodes everything about the geometry). All the crazy time dilation and distance contraction effects you've probably heard about are encoded in this formula.
Today I want to talk about the symmetries of spacetime. What I mean by a symmetry is this: a way to change the coordinates 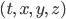 of spacetime in a way that leaves the laws of physics the same. Now I haven't told you what the laws of physics are, but the important thing is that they depend on the geometry of spacetime. So that means that we need to check in what ways we can change the coordinates of spacetime without changing the formula for
of spacetime in a way that leaves the laws of physics the same. Now I haven't told you what the laws of physics are, but the important thing is that they depend on the geometry of spacetime. So that means that we need to check in what ways we can change the coordinates of spacetime without changing the formula for 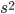 .
.
The first kind of symmetry is called a translation. This consists of simply shifting the coordinate system e.g. one meter to the right, or one second to the future. This doesn't affect the formula for 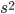 since it only depends on the coordinate differences
since it only depends on the coordinate differences  ,
, 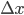 etc. We can write a time translation like this:
etc. We can write a time translation like this:
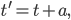
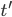 equals the old one plus some number
equals the old one plus some number 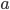 . Similarly, the three possible kinds of spatial translations are:
. Similarly, the three possible kinds of spatial translations are: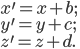
The second kind of symmetry is more complicated, but you've certainly heard of it before—it's called a rotation. If we have two spatial coordinates, then we can rotate them by some angle 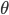 (measured in radians), which leaves all the distances the same. The algebraic formula for a rotation looks like this:
(measured in radians), which leaves all the distances the same. The algebraic formula for a rotation looks like this:
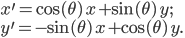
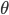 to be a really tiny parameter
to be a really tiny parameter 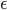 , and just consider the resulting infinitesimal coordinate changes
, and just consider the resulting infinitesimal coordinate changes 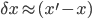 :
: 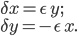
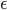 is negative). I'm too lazy to draw this, but if for some reason you can't visualize it, a little bit of fidgeting with any rigid flat object should convince you.
is negative). I'm too lazy to draw this, but if for some reason you can't visualize it, a little bit of fidgeting with any rigid flat object should convince you.
Now actually we have three different spatial coordinates: x, y, and z. That means that you can actually rotate in 3 different ways: along the x-y plane, the y-z plane, and the z-x plane. Of course there are other angles you can rotate at as well, but they are all just combinations of those three; in other words the space of possible rotations is 3-dimensional.
But now, what about the time direction? It would feel terribly lonely if it were left out, and in fact it is also possible to rotate spacetime about the t-x plane, the t-y plane, and the t-z plane. However, remember how time is not quite the same as space? Instead, it's just like space except for a funny minus sign. So not surprisingly, the formula for a rotation also has a funny minus sign—or rather, a funny absence of a minus sign:
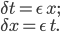
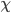 :
: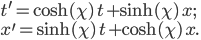
So this rotation has some wierd properties: It describes a crazy world (ours!) in which things rotate in hyperbolas instead of circles. That's because of the minus sign in the formula for 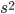 above, which makes it so the points of equal distance (or duration) correspond to hyperbolas instead of circles. This has some additional consequences: 1) Because hyperbolas are infinitely long, the "hyperbolic angle"
above, which makes it so the points of equal distance (or duration) correspond to hyperbolas instead of circles. This has some additional consequences: 1) Because hyperbolas are infinitely long, the "hyperbolic angle" 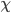 ranges from
ranges from 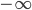 to
to 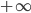 , unlike circular angles which come back to where you started after you rotate through
, unlike circular angles which come back to where you started after you rotate through 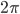 radians. 2) Because the two axes both move towards (or both move away) from each other, when you do a really big rotation it scrunches everything up towards
radians. 2) Because the two axes both move towards (or both move away) from each other, when you do a really big rotation it scrunches everything up towards 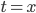 or
or 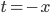 . What this means is that when you accelerate objects more and more, they don't go arbitrarily fast. Instead they just get closer and closer to the speed of light.
. What this means is that when you accelerate objects more and more, they don't go arbitrarily fast. Instead they just get closer and closer to the speed of light.
In conclusion, spacetime has 10 kinds of symmetry: 4 kinds of translations and 6 kinds of rotations. The space of possible symmetries is 10 dimensional. It is called the Poincaré group.
P.S. In this whole discussion I have ignored the possibility of reflection symmetries such as 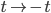 or
or 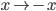 . These are also symmetries of the formula for
. These are also symmetries of the formula for 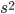 , but they are discrete rather than continuous—there's no such thing as a "small" reflection the way you can have a small rotation. Adding these in doesn't change the fact that the Poincare group is 10 dimensional. However, these transformations are actually NOT symmetries of Nature. They are violated by our theory of the weak force. The only discrete symmetry like this which is preserved by the weak force is CPT: the combination of time reflection, space reflection, and switching matter and antimatter.
, but they are discrete rather than continuous—there's no such thing as a "small" reflection the way you can have a small rotation. Adding these in doesn't change the fact that the Poincare group is 10 dimensional. However, these transformations are actually NOT symmetries of Nature. They are violated by our theory of the weak force. The only discrete symmetry like this which is preserved by the weak force is CPT: the combination of time reflection, space reflection, and switching matter and antimatter.

Pingback: Monday/Tuesday Highlights | Pseudo-Polymath
Pingback: Stones Cry Out - If they keep silent… » Things Heard: e245v1n2
Wait...does this mean that acceleration is a kind of rotation?
Yes, in roughly the same sense that a curved road is a kind of rotation. That is, the vector parallel to the path is rotated at one place, compared to another place.